The Pythagorean Dilemma
Religion can sustain itself only when it is in alignment with either science or the sword. This is the problem the Pythagoreans faced when their numerology ran headlong into the equals sign. An obvious example of this is Antiphon’s calculation of pi. By inscribing and circumscribing a circle with polygons with progressively increasing numbers of sides, he was able to estimate pi to be 3.14—not bad for someone who was tinkering with the first vestiges of calculus. However, no matter how adept they became at calculating areas of polygons, these results inevitably resulted in rational (able to be expressed as a fraction) numbers.
Unfortunately, irrational numbers—numbers that continue after the decimal place forever without repeating themselves—were beyond their skill, not to mention antithetical to their concept of a perfect universe. For all their genius, the geometry of the Greeks is simply insufficient for calculating pi in a meaningful way.
In the 3rd century B.C.E. Eratosthenes was able to calculate the circumference of the Earth to within 0.16 of the modern value, as well as an approximation of the circumference of the Earth using the geometry of triangles. However, without an accurate method for calculating pi his accuracy inevitably hit a wall. Aristarchus, impressive as his calculations of the size and distance of the moon and the sun were using triangle geometry, was never able to apply the scalpel of trigonometry because it required the same mathematical techniques as calculating pi.
The knowledge gap that resulted in a dissonant universe created the same problems with harmony. It would not be until the Medusa of irrational numbers was beheaded that both of her Gorgon sisters would fall.
The Pythagoreans already knew the mathematics of music broke down, if nothing else by the sheer obviousness of the pitches. Philolaus’ ratios were a convenience for finding a few obvious notes, but a system that generated an entire scale required additional effort.
The standard tuning note for an orchestra today is, generally, A440, meaning the string vibrated 440 times per seconds. Obviously this was all Greek to the, well, Greeks. When the Pythagoreans divided that string in half, A440 became A880. A string divided in half vibrates twice as fast, hence 2:1 for an octave. However, as pleasing as this is, the octave doesn’t provide a method of discovering ratios for other pitches. Their fifth—3:2—does.
A440 multiplied by 3:2 yields an E660—a perfect fifth. What exactly does “perfect” mean? It has to do with something called interference.
Interference
Interference is a fancy word for how out of tune something sounds. If you have a string pitched at A440, and another at A445, they don’t sound very pleasing. The reason is that, when the two waves intermingle, sometimes they reinforce each other and sometimes they don’t. To find out how many times per second there is a little bump in volume (wah, wah, wah, wah, wah) you just subtract 445 from 440. Therefore, when those two notes are played together there are five “beats” per second.
We call this “out of tune”, and when the waves are in perfect sync so there are no displeasing bumps we call it “in tune”. It sounds "perfect". The farther apart the notes, the more beats per second, e.g. the more it starts to sound like a lawn mower instead of two good musicians.
So why is A440 and E660 considered a “perfect” interval? Simple. 660–440=220. 220 is half of 440, and therefore the interference wave is an A one octave below 440. In other words, all you hear is another perfectly in tune A. Hooray for math, and the existence of a universal “harmony” has manifested itself before our very eyes.
The Disaster
Being both unafraid of big numbers and dedicated to ratio and proportion, they directed their tonal efforts towards using what they knew to be simple and perfect to try to discover the rest of the tones imbued by the universe with an exact and pleasing ratio. Using today’s notation, if we start on A and go five notes (3:2) we land on E. Five more and we’re on B. Then F#, C#, and so on until you get back to A. Voila. You end up with all twelve notes of the modern chromatic scale.
There’s just one tiny problem. It works in the brain but not in practice. By using math we can see exactly what they heard when they ran it all the way through. Obviously you have to divide the pitches 2:1 to drop them down into the same octave to form a scale, and the numbers in parenthesis are what the Pythagoreans expected to find with their magic ratios.
The D can be ignored as they would have just substituted it for 4:3. However, the C# sounds really bad. In fact, it's so out of tune that writing like simply isn't possible. Worst of all though, math gave them an octave that was horrifically out of tune as well. The C# could be accepted. What should be a perfect 2:1 could not. The substitution was made, but in the back of their minds they knew something was terribly, terribly wrong.
So although we can give the Greeks credit for discovering the embryo of our modern tonal system, they were simply out to sea when it came to how to codify and standardize it into something functional for, say, Bach. Therefore, they made a silk purse. The Greek tonal system had a lot of different rules for which notes should be out of tune for tragedy, comedy, and many other emotions. These are known today as the Greek Modes.
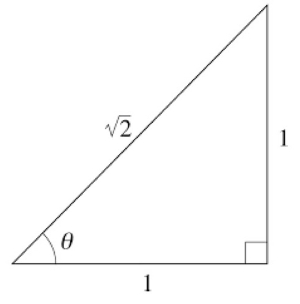
The reason for their failure comes from the simplest irrational number in geometry: √2 . Like pi, the number that screwed up the universe, √2 requires the same unavailable techniques. This number was not unknown to the Pythagoreans either, and was a source of tremendous consternation as it manifests so obviously in a particular kind of triangle: the triangle that divides a square in half.
Using the Pythagorean Theorem (A^2+B^2=C^2) we end up with 1^2 + 1^2 = 2 (the 2 is C squared.
Therefore, the length of the hypotenuse is √2.
This is exactly the number you need to be able to calculate to solve the problem of the failed Pythagorean tuning and unlock Bach's equal temperament.
Nobody in the west would figure this out for centuries, and therefore, the next advance in music would be the attempt by Ptolemy in the 1st c. C.E. to perfect the Greek ratios for the heavens and music, and thereby unlock the mystery of the divine music of the spheres. Ptolemy's model of the heavens and creation of a tuning system known as Just Intonation would be the gold standard of astronomical and musical thought for 1500 years.